One to One Functions - Graph, Examples | Horizontal Line Test
What is a One to One Function?
A one-to-one function is a mathematical function whereby each input corresponds to only one output. In other words, for each x, there is a single y and vice versa. This means that the graph of a one-to-one function will never intersect.
The input value in a one-to-one function is noted as the domain of the function, and the output value is the range of the function.
Let's study the images below:
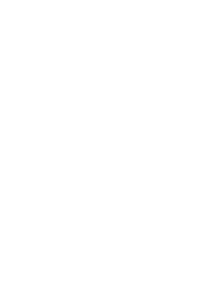
For f(x), every value in the left circle corresponds to a unique value in the right circle. In the same manner, any value in the right circle corresponds to a unique value on the left. In mathematical jargon, this signifies every domain has a unique range, and every range has a unique domain. Therefore, this is an example of a one-to-one function.
Here are some different examples of one-to-one functions:
-
f(x) = x + 1
-
f(x) = 2x
Now let's study the second picture, which exhibits the values for g(x).
Be aware of the fact that the inputs in the left circle (domain) do not own unique outputs in the right circle (range). Case in point, the inputs -2 and 2 have identical output, that is, 4. In the same manner, the inputs -4 and 4 have identical output, i.e., 16. We can discern that there are identical Y values for numerous X values. Hence, this is not a one-to-one function.
Here are different examples of non one-to-one functions:
-
f(x) = x^2
-
f(x)=(x+2)^2
What are the characteristics of One to One Functions?
One-to-one functions have the following properties:
-
The function holds an inverse.
-
The graph of the function is a line that does not intersect itself.
-
The function passes the horizontal line test.
-
The graph of a function and its inverse are the same regarding the line y = x.
How to Graph a One to One Function
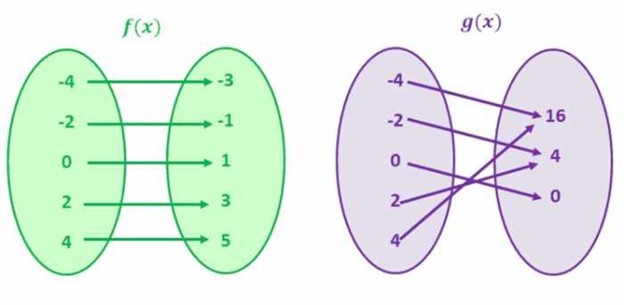
When trying to graph a one-to-one function, you will have to find the domain and range for the function. Let's examine an easy representation of a function f(x) = x + 1.
Once you possess the domain and the range for the function, you ought to plot the domain values on the X-axis and range values on the Y-axis.
How can you evaluate whether a Function is One to One?
To prove whether a function is one-to-one, we can leverage the horizontal line test. As soon as you chart the graph of a function, trace horizontal lines over the graph. If a horizontal line intersects the graph of the function at more than one spot, then the function is not one-to-one.
Since the graph of every linear function is a straight line, and a horizontal line will not intersect the graph at more than one point, we can also reason that all linear functions are one-to-one functions. Keep in mind that we do not leverage the vertical line test for one-to-one functions.
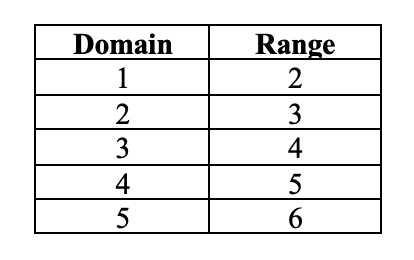
Let's look at the graph for f(x) = x + 1. Once you chart the values for the x-coordinates and y-coordinates, you need to examine if a horizontal line intersects the graph at more than one spot. In this example, the graph does not intersect any horizontal line more than once. This indicates that the function is a one-to-one function.
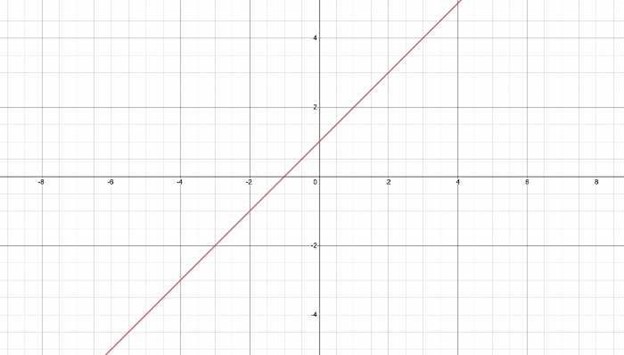
On the contrary, if the function is not a one-to-one function, it will intersect the same horizontal line more than once. Let's look at the diagram for the f(y) = y^2. Here are the domain and the range values for the function:

Here is the graph for the function:
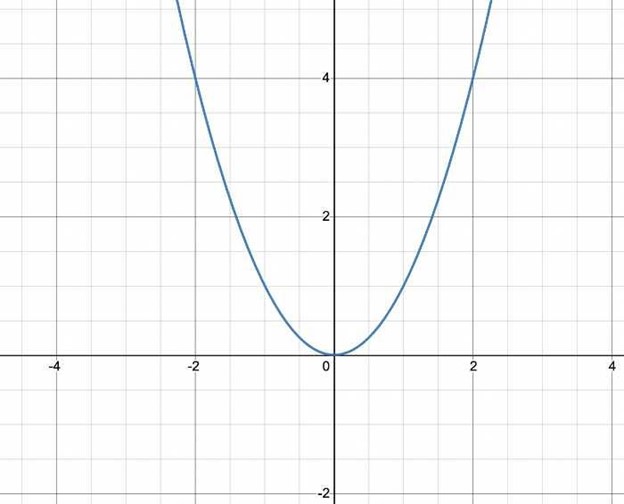
In this instance, the graph crosses numerous horizontal lines. Case in point, for each domains -1 and 1, the range is 1. In the same manner, for both -2 and 2, the range is 4. This implies that f(x) = x^2 is not a one-to-one function.
What is the inverse of a One-to-One Function?
As a one-to-one function has a single input value for each output value, the inverse of a one-to-one function is also a one-to-one function. The inverse of the function essentially undoes the function.
For Instance, in the case of f(x) = x + 1, we add 1 to each value of x in order to get the output, in other words, y. The opposite of this function will deduct 1 from each value of y.
The inverse of the function is denoted as f−1.
What are the characteristics of the inverse of a One to One Function?
The properties of an inverse one-to-one function are the same as any other one-to-one functions. This means that the reverse of a one-to-one function will have one domain for each range and pass the horizontal line test.
How do you figure out the inverse of a One-to-One Function?
Finding the inverse of a function is not difficult. You simply have to swap the x and y values. For example, the inverse of the function f(x) = x + 5 is f-1(x) = x - 5.
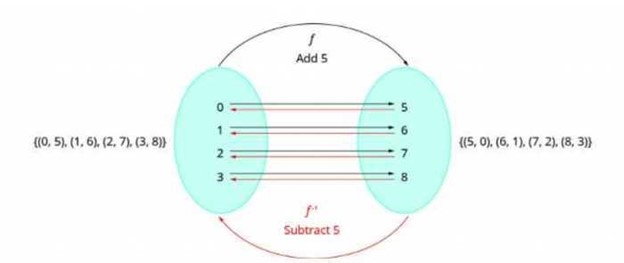
Just like we learned previously, the inverse of a one-to-one function undoes the function. Since the original output value required adding 5 to each input value, the new output value will require us to subtract 5 from each input value.
One to One Function Practice Examples
Consider the subsequent functions:
-
f(x) = x + 1
-
f(x) = 2x
-
f(x) = x2
-
f(x) = 3x - 2
-
f(x) = |x|
-
g(x) = 2x + 1
-
h(x) = x/2 - 1
-
j(x) = √x
-
k(x) = (x + 2)/(x - 2)
-
l(x) = 3√x
-
m(x) = 5 - x
For each of these functions:
1. Determine whether the function is one-to-one.
2. Graph the function and its inverse.
3. Find the inverse of the function numerically.
4. Indicate the domain and range of every function and its inverse.
5. Use the inverse to determine the value for x in each formula.
Grade Potential Can Help You Learn You Functions
If you happen to be struggling trying to understand one-to-one functions or similar functions, Grade Potential can put you in contact with a one on one teacher who can support you. Our New Rochelle math tutors are skilled professionals who assist students just like you advance their mastery of these types of functions.
With Grade Potential, you can learn at your individual pace from the convenience of your own home. Book a call with Grade Potential today by calling (914) 533-1729 to find out more about our educational services. One of our team members will call you to better ask about your needs to provide you with the best teacher for you!